Research Methodology - Statistical Analysis
PROBABILITY - Statistical Analysis
Posted On :
If an experiment is repeated under essentially homogeneous and similar conditions, two possible conclusions can be arrived.
PROBABILITY
If an experiment is repeated under essentially homogeneous and similar conditions, two possible conclusions can be arrived. They are: the results are unique and the outcome can be predictable and result is not unique but may be one of the several possible outcomes. In this context, it is better to understand various terms pertaining to probability before examining the probability theory. The main terms are explained as follows:
An experiment which can be repeated under the same conditions and the outcome cannot be predicted under any circumstances is known as random experiment. For example: An unbiased coin is tossed. Here we are not in a position to predict whether head or tail is going to occur. Hence, this type of experiment is known as random experiment.
A set of possible outcomes of a random experiment is known as sample space. For example, in the case of tossing of an unbiased coin twice, the possible outcomes are HH, HT, TH and TT. This can be represented in a sample space as S= (HH, HT, TH, TT).
Any possible outcomes of an experiment are known as an event. In the case of tossing of an unbiased coin twice, HH is an event. An event can be classified into two. They are: (a) Simple events, and (ii) Compound events. Simple event is an event which has only one sample point in the sample space. Compound event is an event which has more than one sample point in the sample space. In the case of tossing of an unbiased coin twice HH is a simple event and TH and TT are the compound events.
A and A’ are the complementary event if A’ consists of all those sample point which is not included in A. For instance, an unbiased dice is thrown once. The probability of an odd number turns up are complementary to an even number turns up. Here, it is worth mentioning that the probability of sample space is always is equal to one. Hence, the P (A’) = 1 - P (A).
A and B are the two mutually exclusive events if the occurrence of A precludes the occurrence of B. For example, in the case of tossing of an unbiased coin once, the occurrence of head precludes the occurrence of tail. Hence, head and tail are the mutually exclusive event in the case of tossing of an unbiased coin once. If A and B are mutually exclusive events, then the probability of occurrence of A or B is equal to sum of their individual probabilities. Symbolically, it can be presented as:
P (A U B) = P (A) + P (B)
If A and B is joint sets, then the addition theorem of probability can be stated as:
P (A U B ) = P(A) + P(B) - P(AB)
A and B are the two independent event if the occurrence of A does not influence the occurrence of B. In the case of tossing of an unbiased coin twice, the occurrence of head in the first toss does not influence the occurrence of head or tail in the toss. Hence, these two events are called independent events. In the case of independent event, the multiplication theorem can be stated as the probability of A and B is the product of their individual probabilities. Symbolically, it can be presented as:-
P (A B) = P (A) * P (B)
Let A and B be the two mutually exclusive events, then the probability of A or B is equal to the sum of their individual probabilities. (for detail refer mutually exclusive events)
Let A and B be the two independent events, then the probability of A and B is equal to the product of their individual probabilities. (for details refer independent events)
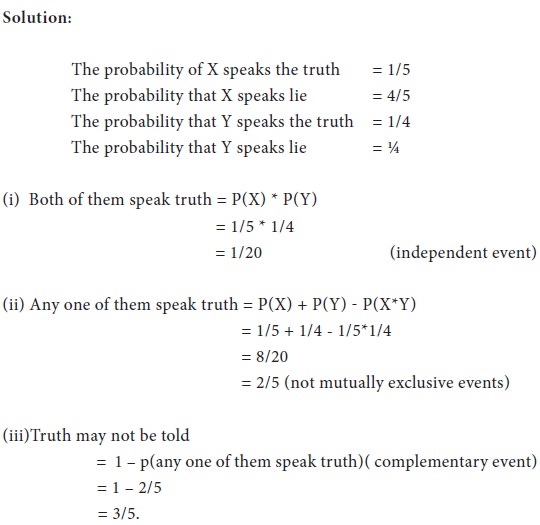
Example: The odds that person X speaks the truth are 4:1 and the odds that Y speaks the truth are 3:1. Find the probability that:-
1. Both of them speak the truth,
2. Any one of them speak the truth and
3. Truth may not be told.
If an experiment is repeated under essentially homogeneous and similar conditions, two possible conclusions can be arrived. They are: the results are unique and the outcome can be predictable and result is not unique but may be one of the several possible outcomes. In this context, it is better to understand various terms pertaining to probability before examining the probability theory. The main terms are explained as follows:
Random Experiment
An experiment which can be repeated under the same conditions and the outcome cannot be predicted under any circumstances is known as random experiment. For example: An unbiased coin is tossed. Here we are not in a position to predict whether head or tail is going to occur. Hence, this type of experiment is known as random experiment.
Sample Space
A set of possible outcomes of a random experiment is known as sample space. For example, in the case of tossing of an unbiased coin twice, the possible outcomes are HH, HT, TH and TT. This can be represented in a sample space as S= (HH, HT, TH, TT).
An Event
Any possible outcomes of an experiment are known as an event. In the case of tossing of an unbiased coin twice, HH is an event. An event can be classified into two. They are: (a) Simple events, and (ii) Compound events. Simple event is an event which has only one sample point in the sample space. Compound event is an event which has more than one sample point in the sample space. In the case of tossing of an unbiased coin twice HH is a simple event and TH and TT are the compound events.
Complementary Event
A and A’ are the complementary event if A’ consists of all those sample point which is not included in A. For instance, an unbiased dice is thrown once. The probability of an odd number turns up are complementary to an even number turns up. Here, it is worth mentioning that the probability of sample space is always is equal to one. Hence, the P (A’) = 1 - P (A).
Mutually Exclusive Events
A and B are the two mutually exclusive events if the occurrence of A precludes the occurrence of B. For example, in the case of tossing of an unbiased coin once, the occurrence of head precludes the occurrence of tail. Hence, head and tail are the mutually exclusive event in the case of tossing of an unbiased coin once. If A and B are mutually exclusive events, then the probability of occurrence of A or B is equal to sum of their individual probabilities. Symbolically, it can be presented as:
P (A U B) = P (A) + P (B)
If A and B is joint sets, then the addition theorem of probability can be stated as:
P (A U B ) = P(A) + P(B) - P(AB)
Independent Event
A and B are the two independent event if the occurrence of A does not influence the occurrence of B. In the case of tossing of an unbiased coin twice, the occurrence of head in the first toss does not influence the occurrence of head or tail in the toss. Hence, these two events are called independent events. In the case of independent event, the multiplication theorem can be stated as the probability of A and B is the product of their individual probabilities. Symbolically, it can be presented as:-
P (A B) = P (A) * P (B)
Addition Theorem of Probability
Let A and B be the two mutually exclusive events, then the probability of A or B is equal to the sum of their individual probabilities. (for detail refer mutually exclusive events)
Multiplication Theorem of Probability
Let A and B be the two independent events, then the probability of A and B is equal to the product of their individual probabilities. (for details refer independent events)
Example: The odds that person X speaks the truth are 4:1 and the odds that Y speaks the truth are 3:1. Find the probability that:-
1. Both of them speak the truth,
2. Any one of them speak the truth and
3. Truth may not be told.

Tags : Research Methodology - Statistical Analysis
Last 30 days 739 views












