Research Methodology - Factor Analysis And Conjoint Analysis
Illustrative Problem - Factor Analysis And Conjoint Analysis
Posted On :
A wrist watch manufacturer desires to find out the combinations of attributes that a consumer would be interested in.
Illustrative Problem
Illustrative Problem 1 : Application Of Rating Scale Technique
A wrist watch manufacturer desires to find out the combinations of attributes that a consumer would be interested in. After considering several attributes, the manufacturer identifies the following combinations of attributes for carrying out marketing research.
Combination – I Mechanism, colour, price, after-scales service
Combination – II Dial, colour, durability, warranty
Combination – III Dial, appearance, price, durability
Combination – IV Mechanism, dial, price, warranty
12 respondents are asked to rate the 4 combinations on the following 3-point rating scale.
Scale – 1 | : | Less important |
Scale – 2 | : | Somewhat important |
Scale – 3 | : | Very important |
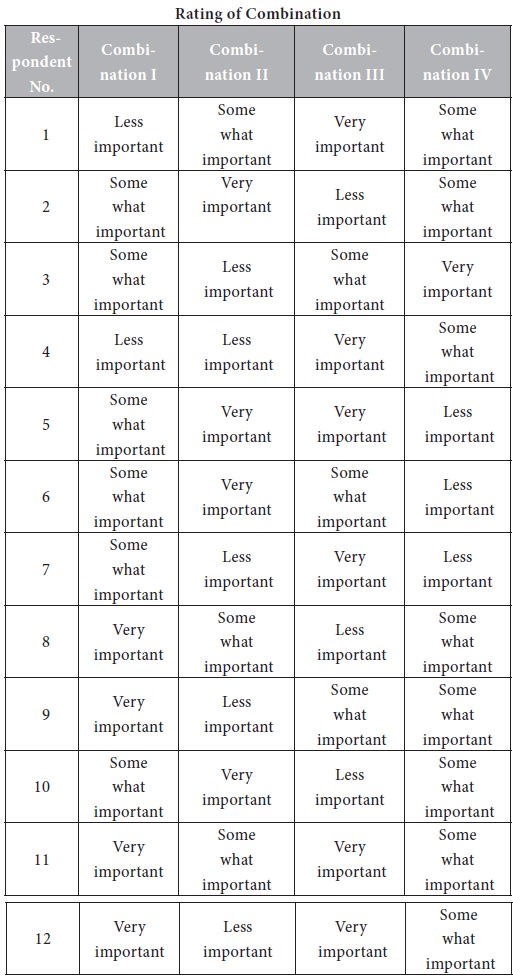
Determine the most important and the least important combinations of the attributes.
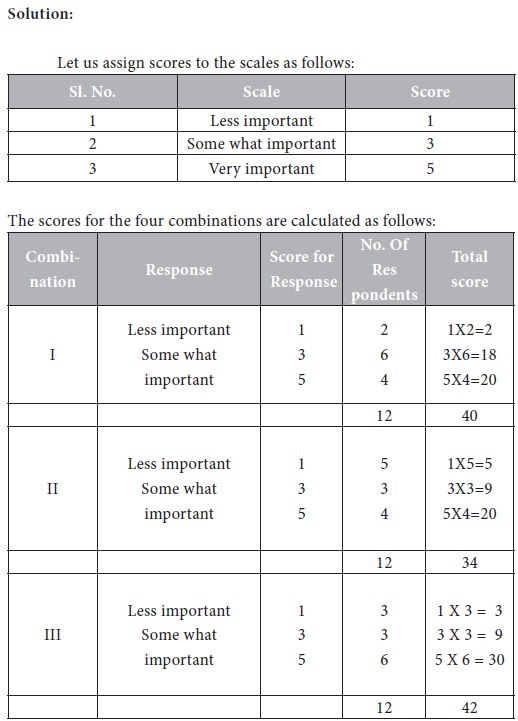
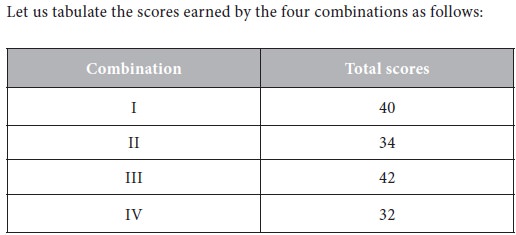
Inference:
It is concluded that the consumers consider combination III as the most important and combination IV as the least important.
Note:
For illustrating the concepts involved, we have taken up 12 respondents in the above problem. In actual research work, we should take a large number of respondents, say 200 or 100. In any case, the number of respondents shall not be less than 30.
Illustrative Problem 2: Application Of Ranking Method
A marketing manager selects four combinations of features of a product for study. The following are the ranks awarded by 10 respondents. Rank one means the most important and rank 4 means the least important.
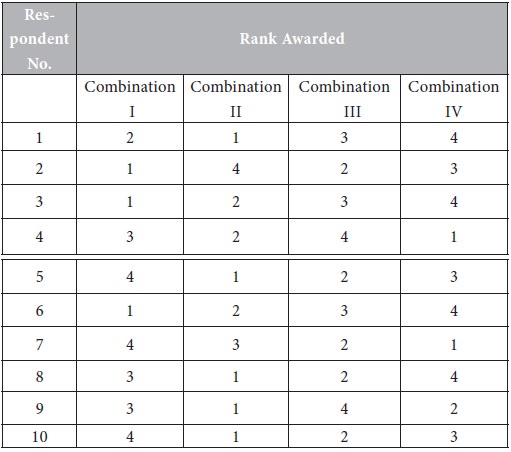
Determine the most important and the least important combinations of the features of the product.
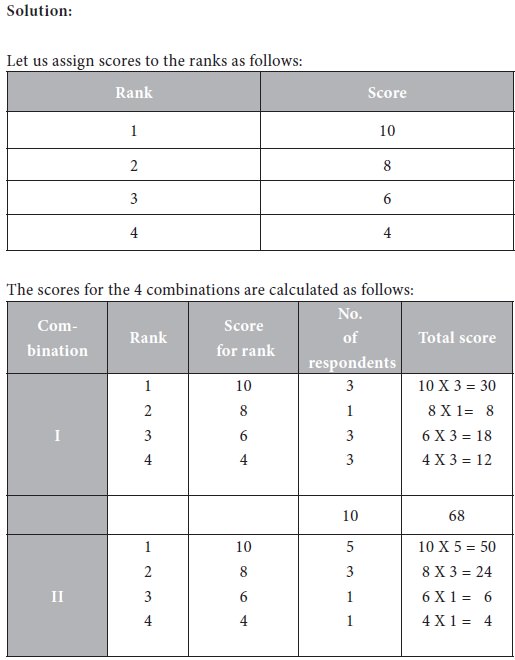
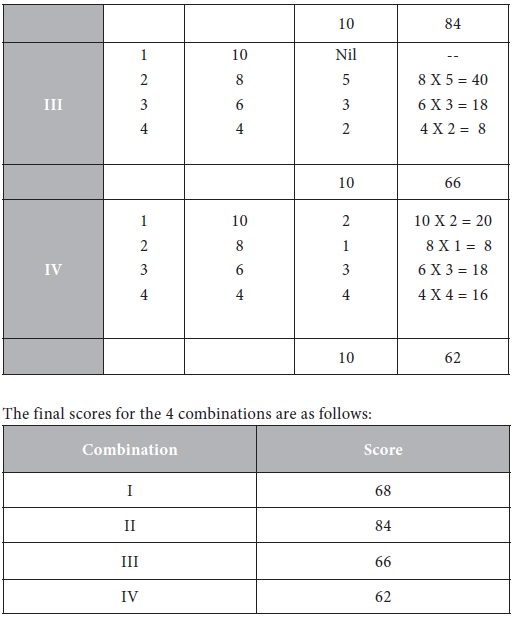
Inference:
It is seen that combination II is the most preferred one by the consumers and combination IV is the least preferred one.
Illustrative Problem 3: Application Of Mini-Max Scaling Method
An insurance manager chooses 5 combinations of attributes of a social security plan for analysis. He requests 10 respondents to indicate their perceptions on the importance of the combinations by awarding the minimum score and the maximum score for each combination in the range of 0 to 100. The details of the responses are given below. Help the manager in the identification of the most important and the least important combinations of the attributes of the social security plan.

Solution:
For each combination, consider the minimum score and the maximum score separately and calculate the average in each case.
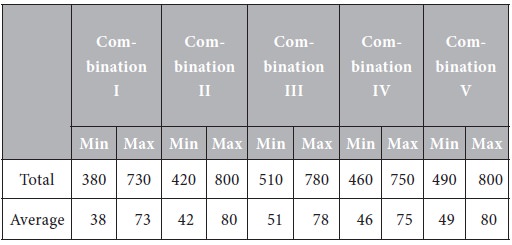
Consider the mean values obtained for the minimum and maximum of each combination and calculate the range for each combination as
Range = Maximum Value – Minimum Value
The measure of importance for each combination is calculated as follows: Measure of importance for a combination of attributes
The measure of importance for each combination is calculated as follows: Measure of importance for a combination of attributes
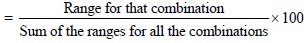
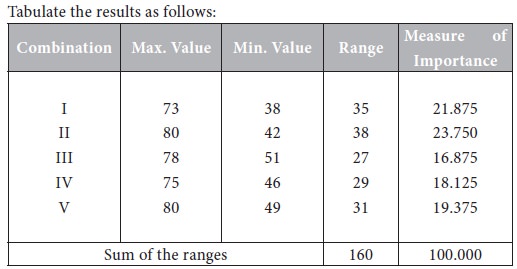
Inference:
It is concluded that combination II is the most important one and combination III is the least important one.
Tags : Research Methodology - Factor Analysis And Conjoint Analysis
Last 30 days 514 views












