Operations Management - Network Problems
Problem of Earliest And Latest Times
Posted On :
The following details are available regarding a project:
Problem
The following details are available regarding a project:

Determine the earliest and latest times, the total float for each activity, the critical activities and the project completion time.
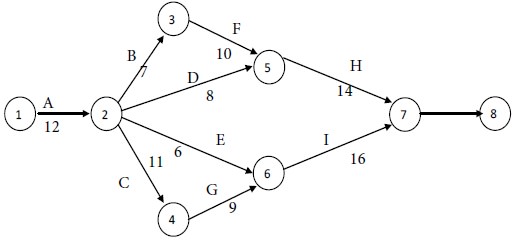
Consider the paths, beginning with the start node and stopping with the end node. There are four such paths for the given project. They are as follows:
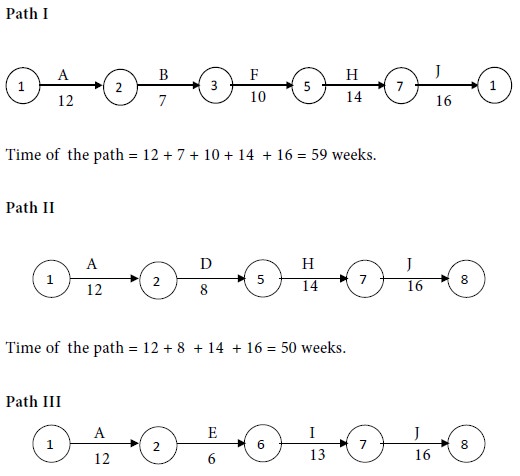
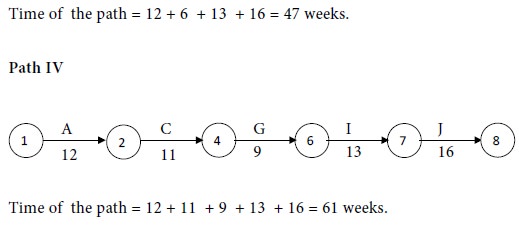
Compare the times for the four paths. Maximum of {51, 50, 47, 61} = 61.
We see that the maximum time of a path is 61 weeks.
Forward pass
Calculation of Earliest Expected Time of Occurrence of Events
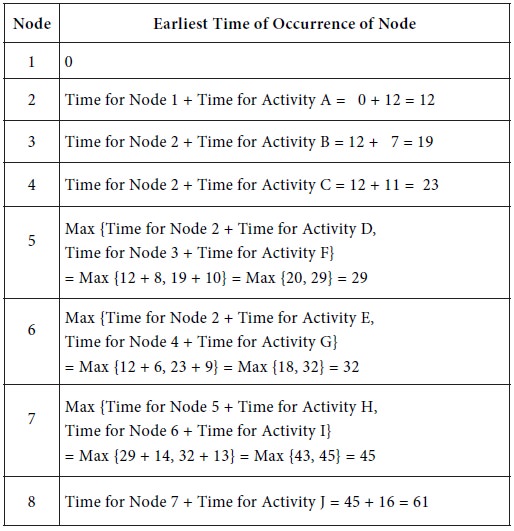
Using the above values, we obtain the Earliest Start Times of the activities as follows:
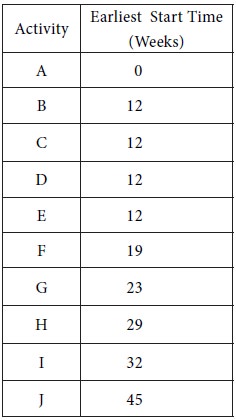
Backward pass
Calculation of Latest Allowable Time of Occurrence of Events
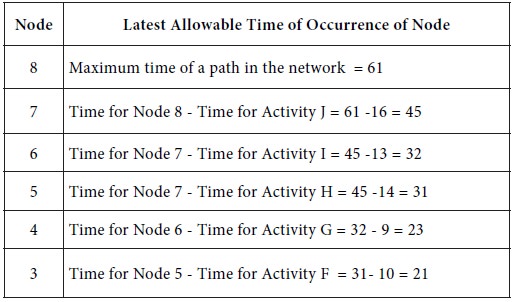
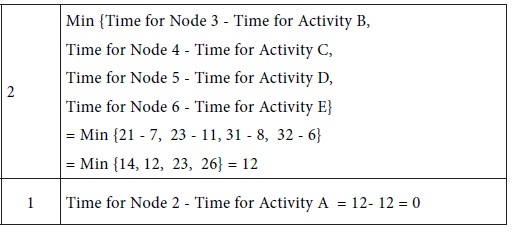
Using the above values, we obtain the Latest Finish Times of the activities as follows:
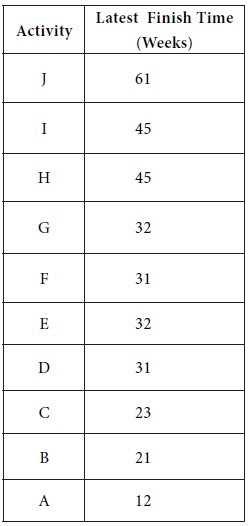
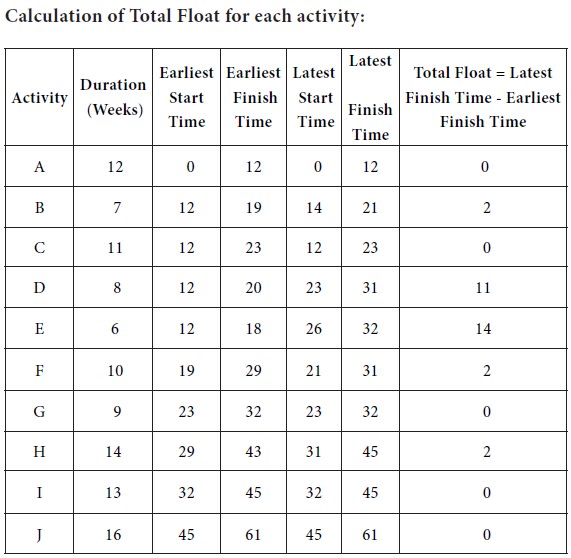
The
activities with total float = 0 are A, C, G, I and J. They are the critical
activities.
Project completion time = 61 weeks.
Problem 2
The following are the details of the activities in a project:
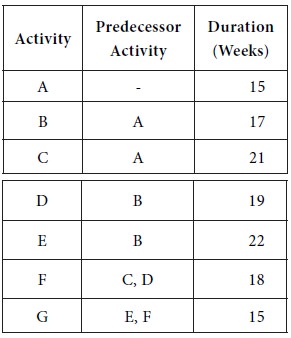
Calculate the earliest and latest times, the total float for each activity and the project completion time.
Solution
The following network diagram is obtained for the given project.
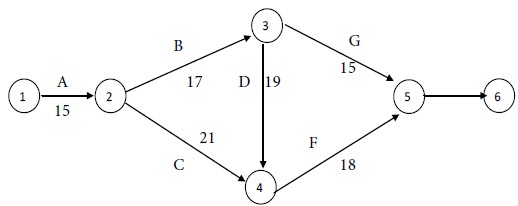
Consider the paths, beginning with the start node and stopping with the end node. There are three such paths for the given project. They are as follows:
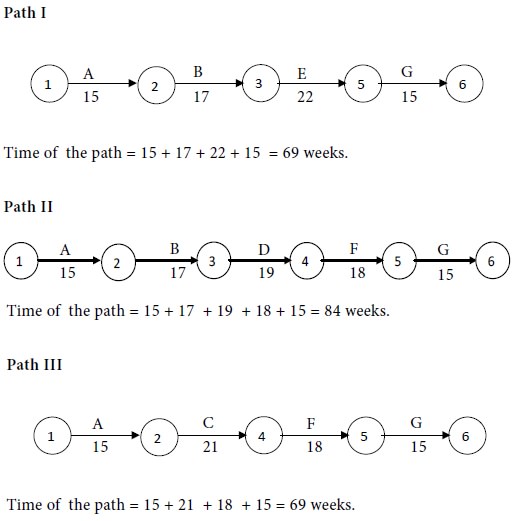
Compare
the times for the three paths. Maximum of {69, 84, 69} = 84. We see that the
maximum time of a path is 84 weeks.
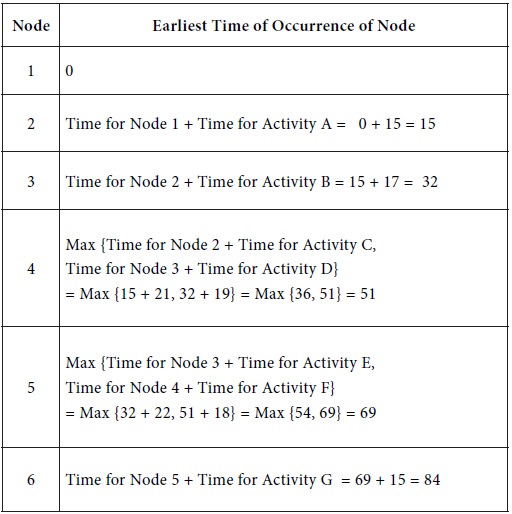
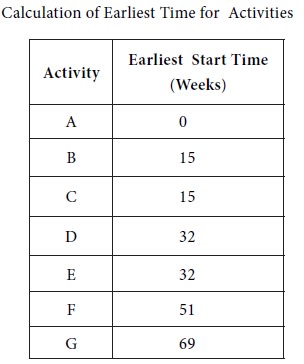
Backward pass
Calculation of the Latest Allowable Time of Occurrence of Events

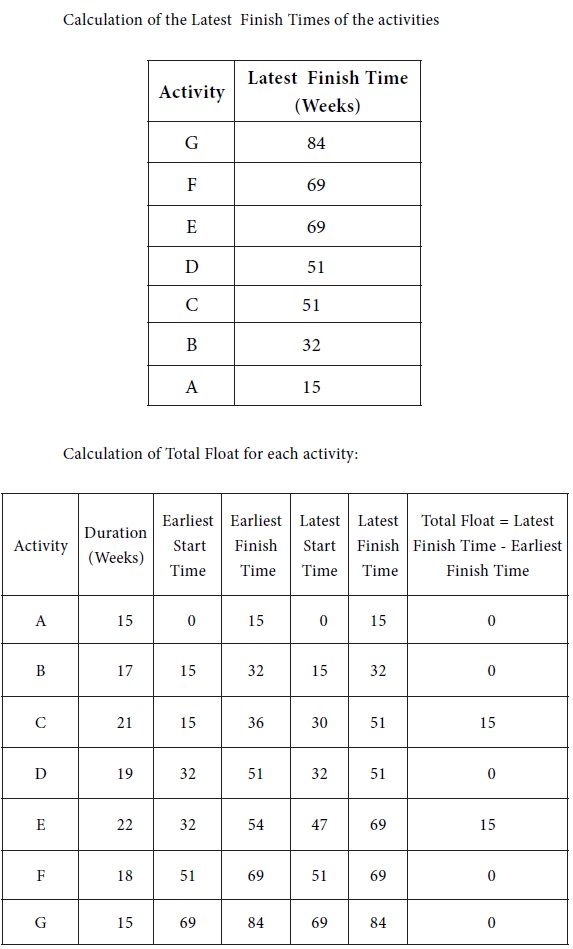
The activities with total float = 0 are A, B, D, F and G. They are the critical activities.
Project completion time = 84 weeks.
Problem 3
Consider a project with the following details:
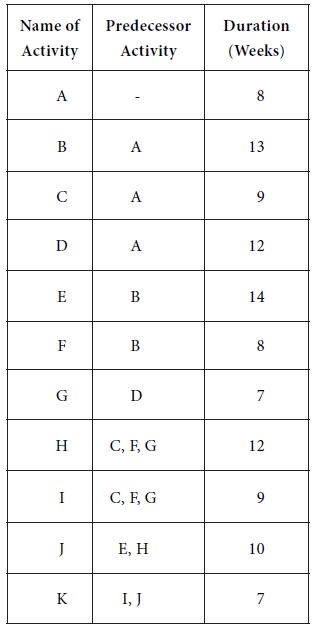
Determine the earliest and latest times, the total float for each activity, the critical activities, the slacks of the events and the project completion time.
Solution
The following network diagram is got for the given project:
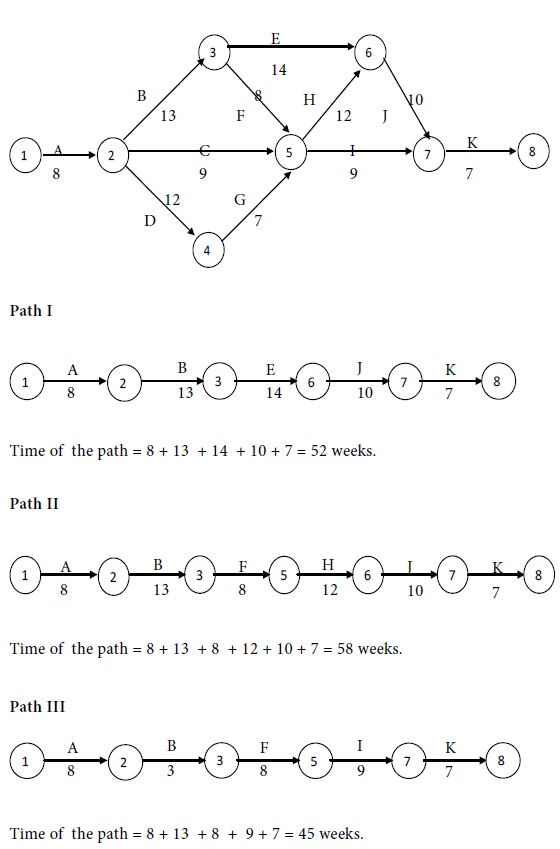
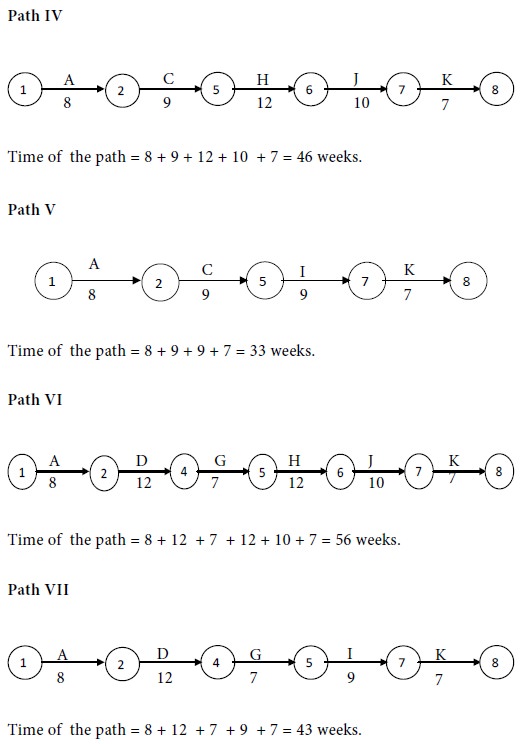
Compare
the times for the three paths. Maximum of {52, 58, 45, 46, 33, 56, 43} = 58.
We see that the maximum time of a path is 58 weeks.
Forward pass
Calculation of Earliest Time of Occurrence of Events
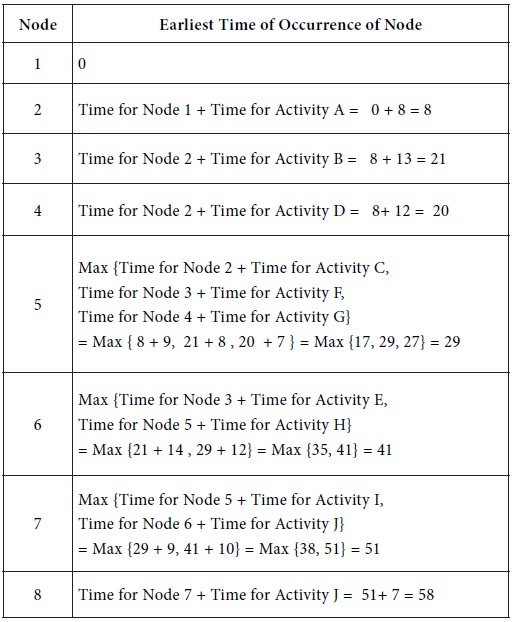
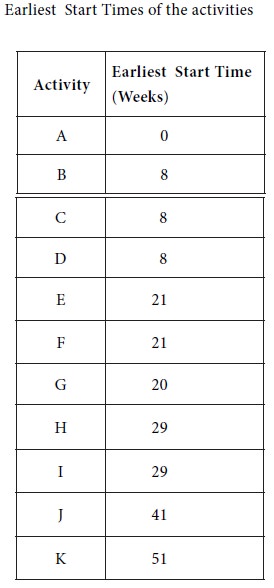
Backward pass
Calculation of Latest Allowable Time of Occurrence of Events
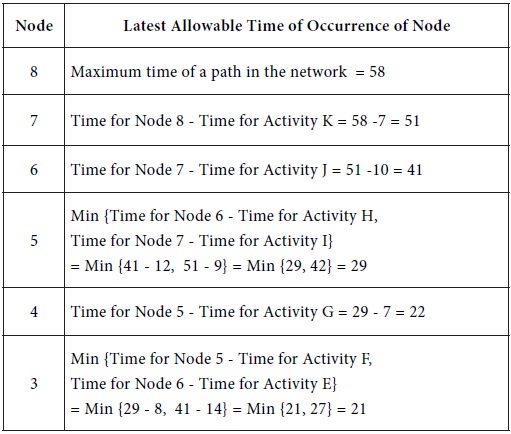
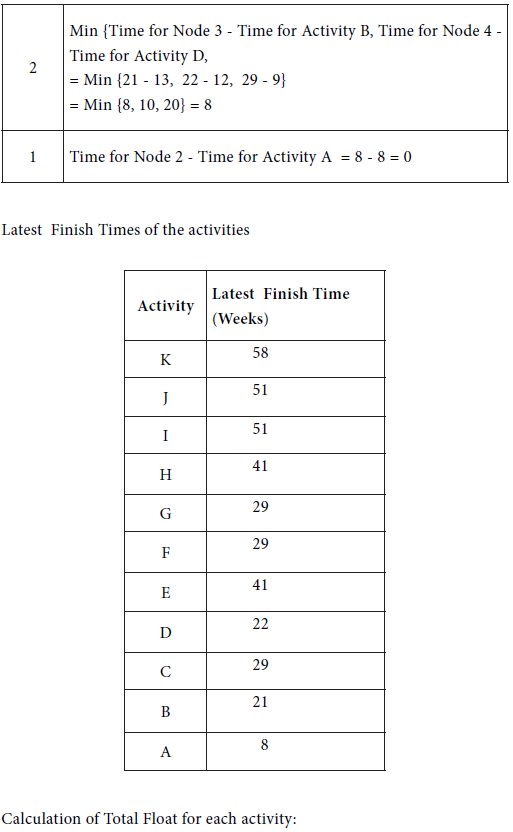
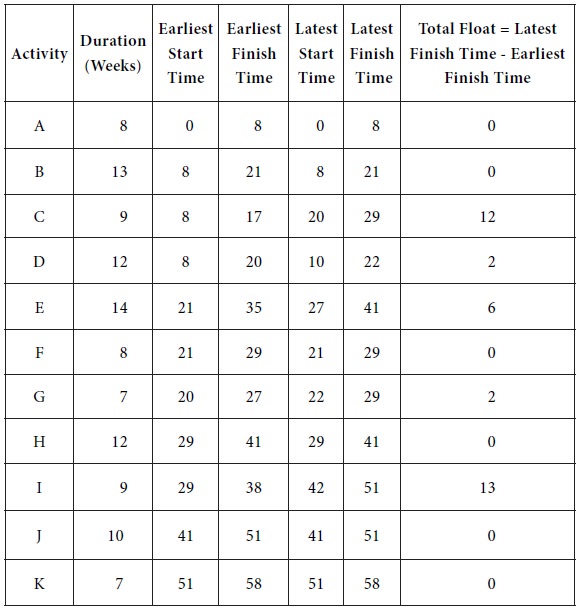
The activities with total float = 0 are A, B, F, H, J and K. They are the critical activities.
Project completion time = 58 weeks.
Calculation of slacks of the events
Slack of an event = Latest Allowable Time of Occurrence of the event - Earliest Expected Time of Occurrence of that event.
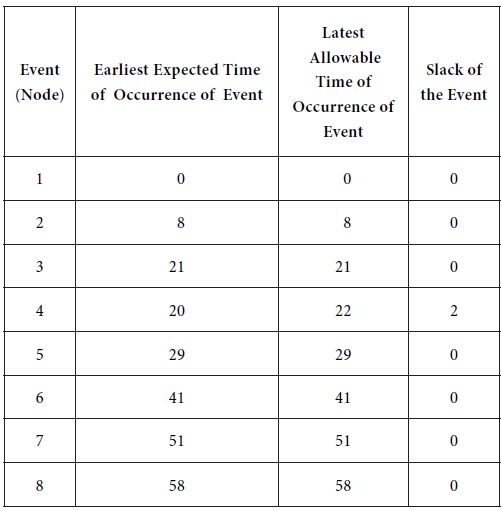
Interpretation
On the basis of the slacks of the events, it is concluded that the occurrence of event 4 may be delayed upto a maximum period of 2 weeks while no other event cannot be delayed.
The following details are available regarding a project:

Determine the earliest and latest times, the total float for each activity, the critical activities and the project completion time.
Solution
With the given data, we construct the following network diagram for the project.
With the given data, we construct the following network diagram for the project.

Consider the paths, beginning with the start node and stopping with the end node. There are four such paths for the given project. They are as follows:


Compare the times for the four paths. Maximum of {51, 50, 47, 61} = 61.
We see that the maximum time of a path is 61 weeks.
Forward pass
Calculation of Earliest Expected Time of Occurrence of Events

Using the above values, we obtain the Earliest Start Times of the activities as follows:

Backward pass
Calculation of Latest Allowable Time of Occurrence of Events


Using the above values, we obtain the Latest Finish Times of the activities as follows:


Project completion time = 61 weeks.
Problem 2
The following are the details of the activities in a project:

Calculate the earliest and latest times, the total float for each activity and the project completion time.
Solution
The following network diagram is obtained for the given project.

Consider the paths, beginning with the start node and stopping with the end node. There are three such paths for the given project. They are as follows:

Forward pass
Calculation of Earliest Time of Occurrence of Events
Calculation of Earliest Time of Occurrence of Events


Backward pass
Calculation of the Latest Allowable Time of Occurrence of Events


The activities with total float = 0 are A, B, D, F and G. They are the critical activities.
Problem 3
Consider a project with the following details:

Determine the earliest and latest times, the total float for each activity, the critical activities, the slacks of the events and the project completion time.
The following network diagram is got for the given project:


We see that the maximum time of a path is 58 weeks.
Forward pass
Calculation of Earliest Time of Occurrence of Events


Backward pass
Calculation of Latest Allowable Time of Occurrence of Events



The activities with total float = 0 are A, B, F, H, J and K. They are the critical activities.
Project completion time = 58 weeks.
Calculation of slacks of the events
Slack of an event = Latest Allowable Time of Occurrence of the event - Earliest Expected Time of Occurrence of that event.

Interpretation
On the basis of the slacks of the events, it is concluded that the occurrence of event 4 may be delayed upto a maximum period of 2 weeks while no other event cannot be delayed.
Tags : Operations Management - Network Problems
Last 30 days 3655 views












